Section 3: Equation Components
The “Unused Portion of K”
Notice that the logistic equation looks like the exponential equation multiplied by [1-(N/K)]. This mathematical term is what accounts for the density-dependent population growth rate.
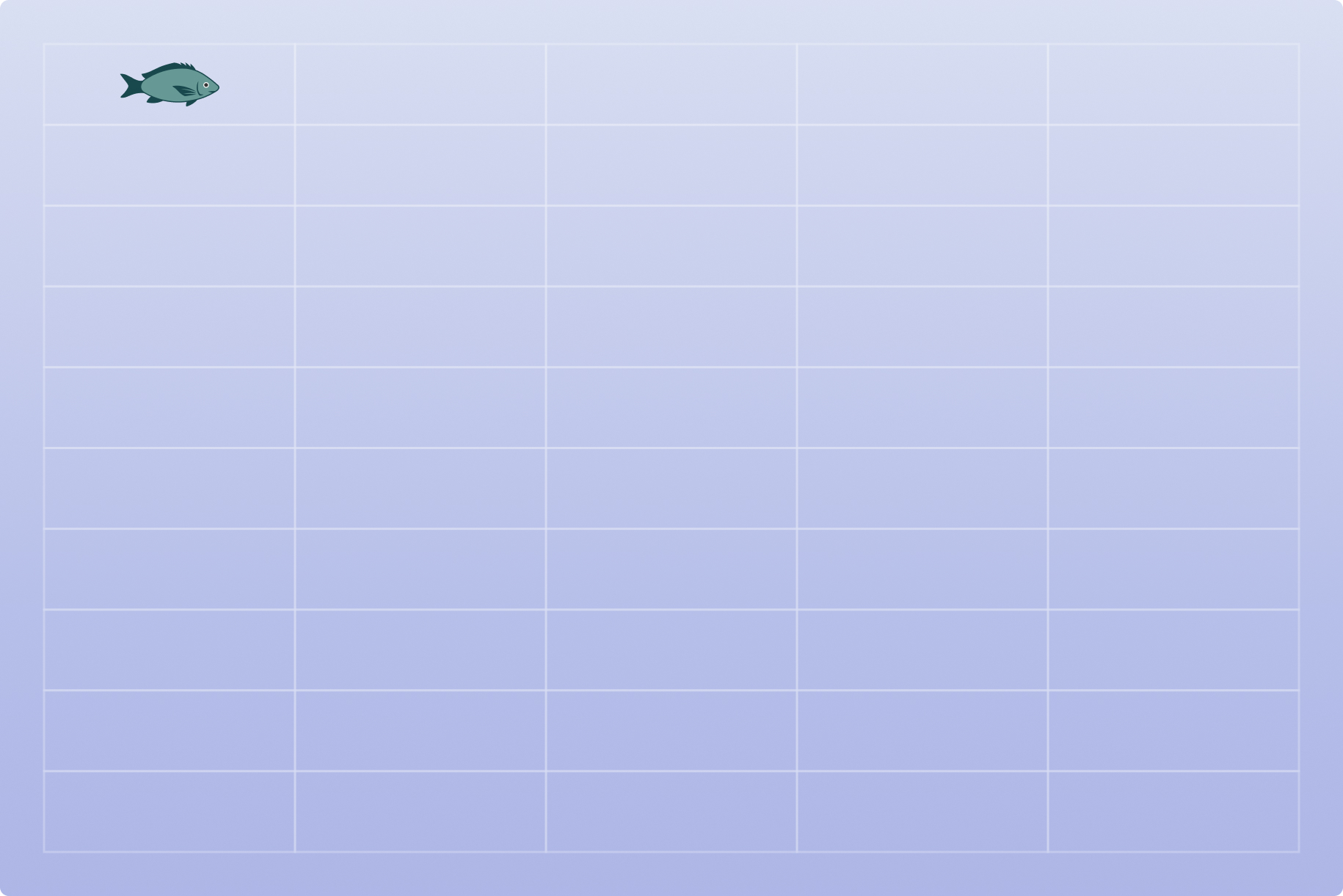
Lake divided into 50 equally sized squares with each square that can only support one fish on average.
Notice that the logistic equation looks like the exponential equation multiplied by [1-(N/K)]. This mathematical term is what accounts for the density-dependent population growth rate.
This gives the per capita growth rate of the population, which is represented as:
The per capita growth rate is mathematically interchangeable with the variable r (realized intrinsic rate of increase). r is the rate of increase at each moment in time. Therefore:
Given this equation for r:
Predict what happens to r as the population reaches K.
If K = 50 and rmax = 0.5, when will r be exactly half of r max? r will be exactly half of rmax when N equals: Number of Fish (N)